neal young / Chrobak06Reverse
-
Information Processing Letters 97:68-72(2006); COCOON'05
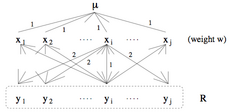 The Reverse Greedy algorithm (RGreedy) for the \(k\)-median
problem works as follows. It starts by placing facilities on
all nodes. At each step, it removes a facility to minimize the
resulting total distance from the customers to the remaining
facilities. It stops when \(k\) facilities remain. We prove that,
if the distance function is metric, then the approximation
ratio of RGreedy is between \(\Omega(\log n/ \log \log n)\) and \(O(\log n)\).Journal version of [2005].
The Reverse Greedy algorithm (RGreedy) for the \(k\)-median
problem works as follows. It starts by placing facilities on
all nodes. At each step, it removes a facility to minimize the
resulting total distance from the customers to the remaining
facilities. It stops when \(k\) facilities remain. We prove that,
if the distance function is metric, then the approximation
ratio of RGreedy is between \(\Omega(\log n/ \log \log n)\) and \(O(\log n)\).Journal version of [2005].
© Copyrights are reserved by the publishers.
Download for personal and limited academic use only.